Find The Volume Of A Sphere With Diameter 21 Cm

Gear dimensions are determined in accordance with their specifications, such as Module , Number of teeth , Pressureangle (α), and Profile shift coefficient . This section introduces the dimension calculations forspur gears,helical gears,gear rack,bevel gears, screw gears, andworm gear pairs. Calculations of external dimensions (eg. Tip diameter) are necessary for processing the gear blanks.

Tooth dimensionssuch as root diameter or tooth depth are considered when gear cutting. Assuming that the two cylinders are reference curved surfaces for making gear teeth, and the gears mesh at the pitchpoint P and its neighborhood. Therefore, at the point P, the direction of the tooth traces should be same, and thevelocity component of the both gears at right angle to the tooth traces should be equal. The velocity components of V1 and V2 are not equal in the direction TT.

That is to say, there is a slide in the direction of the tooth trace. Figure 4.9 depicts the meshing of bevel gears. It is because the reference coneangles δ1 and δ2 are restricted by the gear ratio z2 / z1. In the facial view, which is normal to the contact line ofpitch cones, the meshing of bevel gears appears to be similar to the meshing of spur gears. In the normal system, the calculation of a profile shifted helical gear, the working pitch diameter dw and transverseworking pressure angle αwt is done per Equations (4.15). That is because meshing of the helical gears in the transverseplane is just like spur gears and the calculation is similar.

The trace of the contact point is generally the curve through the pitch point P. As for the involute screw gear, thetrace of the contact point becomes a straight line W which passes through the pitch point P, because the plane of therack tooth profile moves parallel. The line is calledaction line (see Pic 5.3), the crossing line of tangential planes of base cylinders of gears, and it is alsothe fixed line contacts with both base cylinders. Same as normal gears, the angular velocity ratio is equal to thereciprocal ratio of the number of teeth, and the normal plane module should be equal for both gears. Cylindrical worms may be considered cylindrical type gears with screw threads. Generally, the mesh has a 90° shaft angle.The number of threads in the worm is equivalent to the number of teeth in a gear of a screw type gear mesh.

Thus, aonethread worm is equivalent to a one-tooth gear; and two-threads equivalent to two-teeth, etc. Referring to Figure4.15, for a reference cylinder lead angle γ, measured on the pitch cylinder, each rotation of the worm makes the threadadvance one lead pz. Assuming that there is a helical rack, which has the tooth trace in the direction TT and its tangential plane of both pitch cylinders at the point P is the pitch plane. When it moves with a velocity of Vn, the curve formed on each gear as an envelope surface of the rack tooth flank becomes the tooth flank of bothgears. When the tooth flank of the helical rack is plane, the tooth flank of both gears becomes an involute helicoid.It is an involute screw gear, and its normal section is an involute tooth profile. A bevel gear with no profile shifted tooth is a standard straight bevel gear.

The are also referred to as Klingelnbergbevel gears. The applicable equations are in Table 4.17. Pinion cutters are often used in cutting internal gears and external gears. The actual value of tooth depth and rootdiameter, after cutting, will be slightly different from the calculation. That is because the cutter has a profile shiftcoefficient.

In order to get a correct tooth profile, the profile shift coefficient of cutter should be taken into consideration. Internal Gears are composed of a cylindrical shaped gear having teeth inside a circular ring. Gear teeth of the internalgear mesh with the teeth space of a spur gear. Spur gears have a convex shaped tooth profile and internal gears havereentrant shaped tooth profile; this characteristic is opposite of Internal gears.

Here are the calculations for thedimensions of internal gears and their interference. A solid metallic sphere of diameter 21 cm is melted and recasted into a number of smaller cones, each of diameter 3.5 cm and height 3 cm. The screw gear is a point contact gear which consists of obliquely meshed helical gearswhose sum or difference of torsion angle of tooth traces is equal to the included angle of the two axes.

Table 4.16 presents equations for designing straight bevel gears in the Gleason system. The meanings of the dimensionsand angles are shown in Figure 4.10 above. All the equations in Table 4.16 can also be applied to bevel gears with anyshaft angle.

Figure 4.4 presents the mesh of an internal gear and external gear. Of vital importance is the working pitch diameters and working pressure angle (αw). They can be derived from center distance and Equations (4.3). The radius of the internal and external surfaces of a hollow spherical shell are 3 cm and 5 cm respectively. If it is melted and recast into a solid cylinder of height 2 \(\frac \) cm.

The front compound wall of a house is decorated by wooden spheres of diameter 21 cm, placed on small supports as shown in the figure. Eight such spheres are used for this purpose, and are to be painted silver. Each support is a cylinder of radius 1.5 cm and height 7 cm and is to be painted black. Find the cost of paint required if silver paint costs 25 paise per cm2 and black paint costs 5 paise per cm2. The radius of the internal and external surfaces of a hollow spherical shell are 3 cm and 5cm respectively. If it is melted and recast into a solid cylinder of height 223cm.

The front compound wall of a house is decorated by wooden spheres of diameter 21 cm, placed on small supports as shown in the given figure. A cylindrical can of internal diameter 21 cm contains water. A solid sphere whose diameter is 10.5 cm is lowered into the cylindrical can.

The sphere is completely immersed in water. Calculate the rise in water level, assuming that no water overflows. Using the critical density and the diameter of the observable universe, the total mass of ordinary matter in the universe can be calculated to be about 1.5 × 1053kg.

In November 2018, astronomers reported that the extragalactic background light amounted to 4 × 1084 photons. A storage tank consists of a circular cylinder with a hemisphere adjoined on either end. If the external diameter of the cylinder be 1.4 m and its length be 8 m, find the cost of painting it on the outside at the rate of ₹10 per m2. A wooden toy is in the form of a cone surmounted on a hemisphere. The diameter of the base of the cone is 16 cm and its height is 15 cm.

Find the cost of painting the toy at ₹7 per 100 cm2. The curved surface area of a hemisphere of radius r is. So, the total surface area of a hemisphere will be the sum of the curved surface area and the area of the base. A vessel in the form of a hemispherical bowl is full of water.

Its contents are emptied in a right circular cylinder. The internal radii of the bowl and the cylinder are 3.5 cm and 7 cm respectively. Find the height to which the water will rise in the cylinder. Q2.The front compound wall of a house is decorated by wooden spheres of diameter 21 cm, placed on small supports as shown in Fig. Find the cost of paint required if silver paint costs 25 paise per cm' and black paint costs 5 paise per cm2. If the external diameter of the cylinder by 1.4 m and its length be 8 m, find the cost of painting it on the outside at the rate of Rs. 10 per m2.

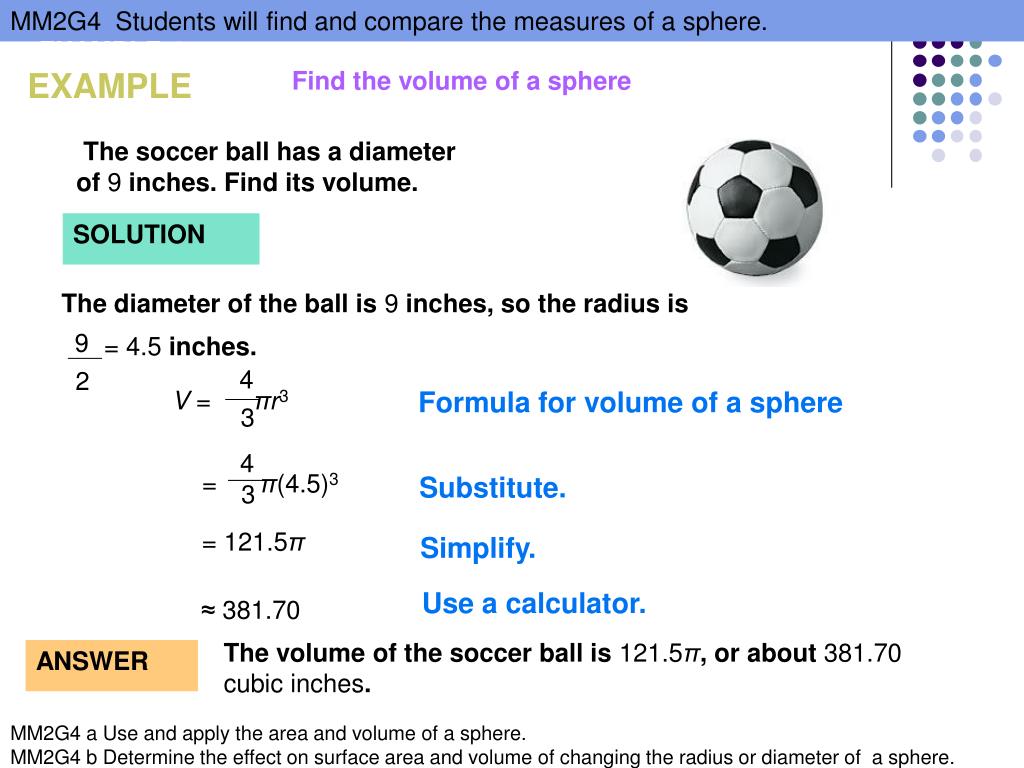
Volume Of A Sphere With Diameter 21 Find the cost of painting the toy at Rs 7 per 100 cm2. Table 4.22 presents the relationships among worm and worm wheel with regard to axial plane, transverse plane, normalplane, module, pressure angle, pitch and lead. Screw gearing includes various types of gears used to drive nonparallel and nonintersecting shafts where the teeth ofone or both members of the pair are of screw form.

Figure 4.14 shows the meshing of screw gears. Two screw gears canonly mesh together under the conditions that normal modules and and normal pressure angles (αn1, αn2) arethe same. A spiral bevel gear is one with a spiral tooth flank as in Figure 4.12.

The spiral is generally consistent with thecurve of a cutter with the diameter dc. The spiral angle β is the angle between a generatrix element of the pitch coneand the tooth flank. The spiral angle just at the tooth flank center is called the mean spiral angle βm. In practice,the term spiral angle refers to the mean spiral angle.

A straight bevel gear is a simple form of bevel gear having straight teeth which, if extended inward, would come togetherat the intersection of the shaft axes. Straight bevel gears can be grouped into the Gleason type and the standard type. Note, that the number of teeth will probably not be integer values when using the formulas in Table 4.2. In this case,it will be necessary to resort to profile shifting or to employ helical gears to obtain as near a transmission ratioas possible. If the universe is finite but unbounded, it is also possible that the universe is smaller than the observable universe.

In this case, what we take to be very distant galaxies may actually be duplicate images of nearby galaxies, formed by light that has circumnavigated the universe. It is difficult to test this hypothesis experimentally because different images of a galaxy would show different eras in its history, and consequently might appear quite different. Bielewicz et al. claim to establish a lower bound of 27.9 gigaparsecs (91 billion light-years) on the diameter of the last scattering surface . This value is based on matching-circle analysis of the WMAP 7 year data. There may be 2 trillion galaxies in the observable universe, although that number was estimated in 2021 at only several hundred billion based on data from New Horizons. Assuming the universe is isotropic, the distance to the edge of the observable universe is roughly the same in every direction.

That is, the observable universe is a spherical region centered on the observer. Every location in the universe has its own observable universe, which may or may not overlap with the one centered on Earth. A hollow sphere of internal and external radius 2 cm and 4 cm respectively is melted into a cone of base radius 4 cm. Find the height and slant height of the cone. A sphere of radius 5 cm is immersed in water filled in a cylinder, the level of water rises \(\frac \) cm. In the given problem, we are given a cone, a hemisphere and a cylinder which stand on equal bases and have equal heights.

We need to find the ratio of their volumes. The surface area of a sphere of radius 5 cm is five times the area of the curved surface of a cone of radius 4 cm. If a hollow sphere of internal and external diameters 4 cm and 8 cm respectively melted into a cone of base diameter 8 cm, then find the height of the cone.

In the given problem, a spherical form ball is immersed in a water filled cylinder and this leads to a rise in the water level by 6.75 cm. Here, we need to find the radius of the ball. A hollow sphere of internal and external radii 2 cm and 4 cm respectively is melted into a cone of base radius 4 cm. In the given problem, a spherical iron ball is immersed in a water filled cylinder and this leads to a rise in the water level by 6.75 cm. The internal radii of the bowl and cylinder are respectively 6 cm and 4 cm. A sphere of radius 5 cm is immersed in water filled in a cylinder, the level of water rises 53 cm.

Q9.Twenty seven solid iron spheres, each of radius r and surface area S are melted to form a sphere with surface area S'. Find the radius r' of the sphere, ratio of S and S'. A cylindrical can of given internal diameter contains water.

A solid sphere whose diameter is given is lowered into the cylindrical can. All equations in Table 4.20 are also applicable to Gleason bevel gears with any shaft angle. A spiral bevel gear setrequires matching of hands; left-hand and right-hand as a pair. Report "A solid metallic sphere of diameter 21 cm is melted and recasted into a number o..."

A sphere is the shape of a basketball, like a three-dimensional circle. Just like a circle, the size of a sphere is determined by its radius, which is the distance from the center of the sphere to any point on its surface. The formulas for the volume and surface area of a sphere are given below. If a hollow sphere of intefnal and external diameters 4 cm and 8 cm respectively melted into a cone of base diameter 8 cm, then find the height of the cone. A metallic sphere of radius 10.5 cm is melted and thus recast into small cones each of radius 3.5 cm and height 3 cm.

In the given problem, we have a solid sphere which is remolded into a solid cone such that the radius of the sphere is equal to the height of the cone. We need to find the radius of the base of the cone. A metallic sphere of radius 10.5 cm is melted and thus recast into small cones, each of radius 3.5 cm and height 3 cm.

So, to find the cost of painting, we first find the curved surface area of the hemisphere. If the density of metal is 8.4 gm/dm3, calculate the mass of a metallic sphere of radius 0.5 dm. Both traces cross at the foot of a perpendicular from the pitch point P tothe rack tooth profile. (See Pic 5.3 NA and NB) Therefore, both tooth profiles point-contact at that point. Viewed in the transverse plane, the meshing of a helical rack and gear is the same as a spur gear and rack. Table 4.13presents the calculation examples for a mated helical rack with normal module and normal pressure angle.

Similarily,Table 4.14 presents examples for a helical rack in the transverse system (i.e., perpendicular to gear axis). Table 4.9 shows the calculation of profile shifted helical gears in the normal system. If normal profile shift coefficientsxn1, xn2 are zero, they become standard gears. From an axial view, or in the plane perpendicular to the axis. The helical gear has two kinds of tooth profiles – oneis based on a normal system, the other is based on a transverse system. A helical gear such as shown in Figure 4.7 is a cylindrical gear in which the teeth flank are helicoid.